Programming Pearls, authored by Jon Bentley, has long been a guiding light for programmers striving for excellence. In this review, we will delve into the 2nd edition of this iconic book and explore the invaluable insights it offers to programmers of all levels.
A Glimpse into the Past
Programming Pearls was first published in 1986 and quickly became a classic in the field of computer science. Jon Bentley, a distinguished computer scientist and software engineer, curated this collection of essays to share his decades of experience with the programming community. Now, with the release of the 2nd edition, Bentley offers updated and expanded wisdom.
Unearthing the Pearls
Problem-Solving Techniques
The book opens with a chapter on problem-solving techniques. Bentley encourages readers to think beyond the obvious solutions and to seek elegant and efficient ways to tackle coding challenges. He emphasizes the importance of understanding the problem deeply before diving into code.
Coding Style and Efficiency
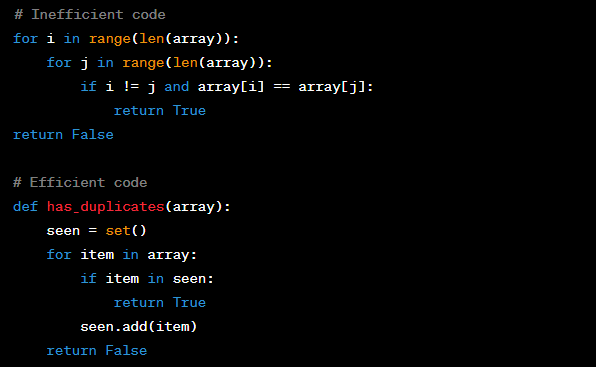
Bentley’s discussion on coding style and efficiency is a gem. He not only guides readers on writing clean and efficient code but also highlights the significance of code readability and maintainability. The examples provided are clear and illustrative.
Example:
Algorithm Design
Bentley dives deep into algorithm design, teaching readers how to think critically about problem-solving and algorithmic efficiency. He uses real-world examples to illustrate complex concepts, making it easier for readers to grasp.
Charting the Course
Let’s take a moment to compare the 1st and 2nd editions of Programming Pearls to see how the book has evolved.
| Aspect | 1st Edition | 2nd Edition |
|---|---|---|
| Content | Classic essays | Updated and expanded |
| Code Examples | C and Pascal | Includes modern languages |
| New Chapters | No | Yes |
| Software Engineering | Limited discussion | Extensive insights |
The Modern Programmer’s Toolkit
In today’s fast-paced tech landscape, Programming Pearls 2nd Edition equips programmers with essential tools for success. Bentley introduces new chapters, including topics on software engineering and modern programming languages.
The Essence of Problem-Solving
In Programming Pearls 2nd Edition, Bentley underscores the essence of problem-solving. He emphasizes the importance of thinking like a detective when faced with coding conundrums. The book encourages programmers to dissect problems into manageable components and iteratively refine solutions.
One of the most valuable takeaways is Bentley’s focus on the psychology of programming. He discusses common pitfalls, such as “off-by-one” errors and the complexities of managing memory, providing practical strategies to overcome these challenges.

Problem-Solving Strategies
To highlight the practical problem-solving approach presented in the book, let’s outline some key strategies:
- Divide and Conquer: Break down complex problems into smaller, solvable parts;
- Brute Force vs. Elegance: Explore both straightforward and elegant solutions, weighing trade-offs;
- Testing and Debugging: Learn efficient debugging techniques to identify and resolve issues.
Mastering Algorithmic Challenges
Programming Pearls 2nd Edition takes readers on a journey through algorithmic challenges that span various domains. Bentley’s ability to elucidate complex algorithms using real-world analogies is a standout feature of the book.
Algorithmic Challenges
| Challenge | Description |
|---|---|
| Sorting Algorithms | Explore various sorting algorithms and their efficiency. |
| String Manipulation | Learn techniques for efficient string manipulation. |
| Searching Algorithms | Dive deep into searching algorithms and their applications. |
Timeless Wisdom for Modern Developers
The enduring appeal of Programming Pearls lies in its ability to bridge the gap between classic programming principles and contemporary software development practices. The 2nd edition, in particular, brings this wisdom into the modern era.
Key Updates in the 2nd Edition
| Aspect | Description |
|---|---|
| Code Examples | Updated to include Python, Java, and more. |
| Software Engineering | Extensive insights into modern practices. |
| New Chapters | Explores software engineering and design patterns. |
| Language Flexibility | Adaptable to multiple programming languages. |
Programming Pearls in Practice
To truly appreciate the value of Programming Pearls, it’s crucial to understand how these insights translate into real-world coding scenarios. Let’s consider a practical example where the book’s principles can be applied.
Example: Optimizing Database Queries
Imagine you’re tasked with optimizing a database query that’s become slow as your application’s data volume has grown. Applying Bentley’s problem-solving approach, you might:
- Analyze the Problem: Break down the query’s execution to identify bottlenecks;
- Consider Multiple Solutions: Explore indexing, caching, or query optimization techniques;
- Testing and Benchmarking: Implement changes and rigorously test for performance improvements.

Practical Code Optimization Techniques
One of the book’s most practical aspects is its focus on code optimization. Bentley recognizes the importance of writing efficient code, not just in terms of algorithmic complexity but also regarding runtime performance.
Key Optimization Strategies
Here are some optimization strategies the book covers:
- Profiling: Bentley explains how to use profiling tools to identify performance bottlenecks in your code;
- Data Structures: Learn how choosing the right data structures can significantly impact program efficiency;
- Caching: Understand the power of caching to reduce redundant computations and database queries;
- Parallelism: Explore techniques for parallelizing code to leverage multi-core processors effectively.
Case Studies: Applying Programming Pearls
To solidify the book’s teachings, Bentley includes several compelling case studies where he applies the concepts discussed. These real-world examples serve as a bridge between theory and practice.
Notable Case Studies
| Case Study | Description |
|---|---|
| Case Study | Description |
| Text Formatting | Creating a text formatter with an efficient algorithm. |
| Binary Search and B-Trees | In-depth exploration of these fundamental data structures. |
Beyond the Book: Community and Resources
The Programming Pearls community is a testament to the book’s enduring influence on the programming world. Bentley’s work has sparked discussions, collaborations, and further exploration of the topics he presents.
Resources for Programmers
- Online Forums: Join discussions on programming forums and communities dedicated to Programming Pearls;
- GitHub Repositories: Explore open-source projects inspired by the book’s principles;
- Coding Challenges: Test your problem-solving skills with coding challenges that draw inspiration from the book.
Conclusion
In conclusion, Programming Pearls 2nd Edition is a must-read for programmers and software engineers at all levels of expertise. It offers timeless wisdom and practical advice, making it a valuable addition to any developer’s bookshelf.
FAQs
Absolutely! While some sections may be more advanced, the book offers valuable insights for programmers of all levels.
Yes, the 2nd edition includes updated examples in modern languages like Python and Java.
The 2nd edition features new chapters, expanded content, and a focus on modern software engineering practices.
Absolutely, the book’s timeless principles and problem-solving techniques remain relevant and valuable.
You can find the book on major online book retailers and in local bookstores.
